Force of Friction | Expression for the acceleration and the work done on inclined rough surface| Advantage and Disadvantage

Definition of Friction:

When an object slides over the surface of another object then each surface applies a parallel force to the other. This force is called friction force. The direction of the force of friction on every object with respect to the other is always opposite to the motion of the second object.

$F=\mu R$

Properties of Friction Force:

The force of friction always acts in the opposite direction of the motion of the object.

The force of friction always opposes the motion of the object. So it does not help in motion.

Friction force always helps an object to be in the rest of the position. i.e If an object is in the rest of the position that is due to only frictional force.

The frictional force is act on both states of the object i.e Rest and motion.

Modern Concept of Friction:

It is now believed that frictional forces arise because of a fundamental force occurring in nature commonly known as an electric force. Every object has surface irregularities at the atomic level.

When two surfaces come in contact then the real area in contact is much smaller than the actual area of the surface. At the contact points, the distance between the particles becomes very small and as such the molecular electrical forces begin to act between the surfaces and molecular bonds are formed.

When one of the surfaces is pulled over the other, the molecular bonds are broken. As a result, the material of the objects is deformed and new bonds are formed. The successive loading and deformation processes result in loss of energy which appears in the form of heat. To compensate for the energy loss, a force is required to be applied to start the motion.

Types of forces of friction: There are two types of forces of friction

1. Force of Static Friction
2. Force of Dynamic Friction

1. Force of Static Friction:

When the friction forces acting between two surfaces at rest with respect to each other are called the force of static friction.

Note: The maximum value of the force of static friction is always equal to the minimum value of force required to start the motion. Once the motion starts, the force of friction after reducing becomes such that it is sufficient to maintain uniform motion.

Due to the force of static friction between two dry and unlubricator surfaces, the maximum force is according to the following two laws:

i.) The maximum force does not normally depend upon the area of contact.
ii.) It is directly proportional to the normal reaction.

Coefficient of Static Friction:

The ratio of the maximum value of force in static friction and normal reaction is called the coefficient of static friction.

$\mu_{s}=\frac{F}{R}$

2. Force of Dynamic Friction:

When the force of friction acting between the two surfaces in relative motion is called the force of dynamic friction.

Note: Due to dynamic friction between the dry and unlubricated surfaces the value of dynamic friction is according to the two laws:

i.) It does not depend upon the surfaces of contact.
ii.) It is equal to the maximum normal reaction force.

Coefficient of Dynamic Friction:

The ratio of force in dynamic friction and normal reaction is called the coefficient of dynamic friction.

$\mu_{k}=\frac{F}{R}$

Limiting friction:

It is the maximum value of friction that acts when the object just begins to move is called Limiting Friction. The limiting friction is directly proportional to the normal reaction in the object. i.e

$F \propto R$

$F = \mu R$

Angle of friction:

The angle between the effective resistance $S$ (i.e resultant of frictional force and normal reaction) and normal reaction $R$ is called the angle of friction.

Let us consider, A object of mass $m$ placed on a horizontal surface. If $F$ force is applied on the object then the value of limiting friction

$F \propto R$

$F = \mu R$

$\mu=\frac{F}{R} \qquad(1.1)$

From figure in $\Delta ROS$

$tan \theta =\frac{RS}{RO}$

$tan \theta =\frac{RS}{RO}$

$tan \theta =\frac{F}{R} \qquad \left( \because RS=F \: and \: RO=R \right)$

From the equation $(1.1)$ and the above equation, We can conclude that

$\mu=tan\theta$

Angle of Repose and Angle of Sliding:

The angle of repose or angle of sliding is defined as the minimum angle of inclination of a surface with the horizontal, such that an object placed on the surface just begins to slide down.

The various forces acting on the object are shown in the figure below:

i.) Weight $mg$ of the object acting vertically downwards.
ii.) Normal Reaction $R$ acting perpendicular to inclined surface $AB$
iii.) Force of friction $f$ acting up in the inclined surface $AB$

Now from the figure apply the equilibrium condition:

$R=mg \: cos\theta \qquad(1.2)$

$f=mg \: sin\theta \qquad(1.3)$

Now divide by equation $(1.3)$ to equation $(1.2)$

$\frac{f}{R}=\frac{mg \: sin\theta}{mg \: cos\theta}$

$\frac{f}{R}=\frac{sin\theta}{cos\theta}$

$\frac{f}{R}=tan \theta$

$\frac{\mu R}{R}=tan \theta \qquad \left( \because f=\mu R \right)$

$\mu =tan \theta $

Thus the angle of friction is equal to the angle of repose.

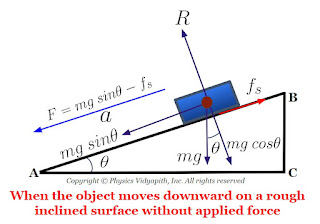
1.) Expression for the acceleration and work done on the object moves downward on a rough inclined surface without applied force:

Let us consider, A object of mass $m$ moving sliding downward on a rough surface that is inclined at an angle $\theta$ from horizontal. If the angle $\theta$ of the inclined surface is greater than the angle of repose, the object slides down with an acceleration $a$ without any applied force.

The various forces acting on the object are shown in the figure below:

i.) Weight $mg$ of the object acting vertically downwards.
ii.) Normal Reaction $R$ acting perpendicular to inclined surface $AB$
iii.) Force of friction $f$ acting up in the inclined surface $AB$

Apply the equilibrium condition from the figure:

$R=mg \: cos\theta \qquad(1.4)$

The net force on the object moving down the inclined surface

$F=mg \: sin\theta - f \qquad(1.5)$

$F=mg \: sin\theta - \mu R $

Now substitute the value of $R$ from equation $(1.4)$ in above equation

$F=mg \: sin\theta - \mu mg \: sin \theta$

$ma=mg \left( \: sin\theta - \mu \: sin \theta \right) \qquad( F=ma)$

$a=g \left( \: sin\theta - \mu \: sin \theta \right) $

If the object is displaced the distance $S$ by the acceleration $a$ then work done by force:

$W=F.S$

$W= mg \left(\: sin\theta - \mu \: sin \theta \right). S$

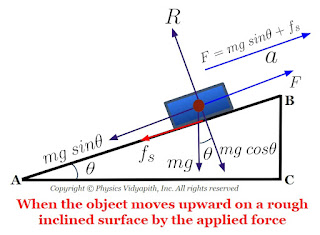
2.) Expression for acceleration and work done on the object moving upward on a rough inclined surface by the applied force:

Let us consider, A object of mass $m$ moving upward on a rough inclined surface by applying the force $F$ sliding downward on a rough surface so the various forces acting on the object are shown in the figure below:

i.) Weight $mg$ of the object acting vertically downwards.
ii.) Normal Reaction $R$ acting perpendicular to inclined surface $AB$
iii.) Force of friction $f$ acting up in the inclined surface $AB$

In equilibrium condition from the figure:

$R=mg \: cos\theta \qquad(1.6)$

The net force on the object moving down the inclined surface

$F=mg \: sin\theta + f \qquad(1.7)$

$F=mg \: sin\theta + \mu R $

Now substitute the value of $R$ from equation $(1.6)$ in above equation

$F=mg \: sin\theta + \mu mg \: sin \theta$

$ma=mg \left( \: sin\theta + \mu \: sin \theta \right) \qquad( \because F=ma)$

$a=g \left( \: sin\theta + \mu \: sin \theta \right) $

If the object is displaced the distance $S$ by the force $F$ then work done by force:

$W=F.S$

$W= mg \left(\: sin\theta + \mu \: sin \theta \right). S$

3.) Expression for acceleration and work done on the object moves on a rough horizontal surface by the applied force:

Let us consider, A object is moving on a rough horizontal surface by applying the force $F$ on the object of mass $m$ and then the net force on the object from the figure below

$F-f=ma$

$F-\mu R=ma \qquad \left(\because f=\mu R \right)$

$F-\mu mg=ma \qquad \left(R=mg \right)$

$F=m \left(\mu g+a \right)$

$a=\frac{F-\mu mg}{m}$

If the object is displaced the distance $S$ by the force $F$ then work done by force:

$W=F.S$

$W= m \left(\mu g+a \right). S$

The Advantages of Friction:

The two objects will not stick to each other if there is no friction between the surface.

The parts of machinery are held together with the help of nuts and bolts but without friction, these can not be held.

A person can not walk or stand on the surface without friction.

The brakes of any vehicle will not work without friction.

It is not possible to transfer motion from one part of a machine to the other part without the help of friction.

Sandpaper is used in cleaning because this cleaning is only possible with the help of friction.

Adhesives will lose their purpose.

The Disadvantage of Friction:

Friction always opposes the relative motion between any two objects in contact. Therefore, extra energy is lost to overcome friction. Thus, friction involves the unnecessary loss of energy. This shows that the output is always less than the input.

Friction between the parts of machinery causes wears and tear. Therefore, the lifetime of the parts of the machinery reduces.

Frictional forces produce heat, which causes damage to the machinery.

Methods of Reducing friction:

By Polishing: Polishing makes the surface smoother. Therefore, friction reduces.

By Streamlining: Friction due t air is considerably reduced by streamlining the shape of the object (sharp in front) moving through the air.

By Lubrication: Oil, grease, and many other materials are used as lubricants. These lubricants fill up the irregularities of the surface making them smother. Hence, friction decreases.

By proper selection of materials.

By using ball bearings.