Derivation of Gravitational Potential due to Point mass and on the Earth

Definition of Gravitational Potential:

When an object is brought from infinity to a point in the gravitational field then work done acquired by the gravitational force is called gravitational potential.

Let us consider, An object of mass $m$ brought from infinity to a point in the gravitational field. If work done acquired by force is $W$ then gravitational potential

$V=-\frac{W}{m}$

Derivation of Gravitational Potential due to a Point mass:

Let us consider,
The mass of the point object (i.e point mass)=$m$
The mass of the object that produces the gravitational field = $M$

If the point mass $m$ is at a distance $x$ then the gravitational force between the objects is

$F=G \frac{M \: m}{x^{2}} \qquad(1)$

If the point mass moves a very small distance element $dx$ that is at distance $x$ from point $O$ then the work done to move the point object from point $B$ to $A$

$dw=F.dx$

Now substitute the value of $F$ from equation $(1)$ in above equation

$dw=G \frac{M \: m}{x^{2}}.dx$

Therefore, the work done to bring the point mass from infinity to point $P$ that is at distance $r$ from point $O$ then work done to move the point object from infinity ($\infty$) to point $P$

$\int_{0}^{W} dw = \int_{\infty}^{r} G \frac{M \: m}{x^{2}}.dx $

$ [w]_{0}^{W} = G\: M\: m \int_{\infty}^{r} \frac{1}{x^{2}}.dx $

$ [W-0] = G\: M\: m [-\frac{1}{x} ]_{\infty}^{r} $

$ W = G\: M\: m [\left(-\frac{1}{r}\right) - \left(-\frac{1}{\infty}\right)]$

$ W = -\frac{G\: M\: m }{r} \qquad \left( \because \frac{1}{\infty} =0 \right)$

$ W = -\frac{G\: M\: m }{r} $

$\frac{W}{m} = -\frac{G\: M }{r} $

$ V = -\frac{G\: M}{r} \qquad \left( \because V=\frac{W}{m} \right)$

$ V = -\frac{G\: M}{r}$

Thus the above equation represents the gravitational potential at point $P$

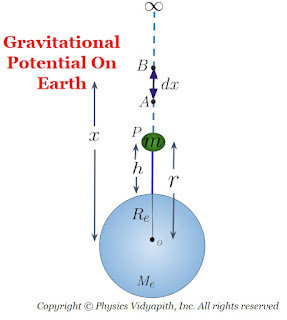
Gravitational Potential on Earth:

Let us consider, The mass of Earth = $M_{e}$
The radius of earth = $R_{e}$
The mass of the object = $m$
The distance from centre $O$ of the earth to point $P$ = $r$
The distance from the surface of the earth to point $P$ = $h$

If the object is at a distance $x$ then the gravitational force is

$F=G \frac{M_{e} \: m}{x^{2}} \qquad(1)$

If the object moves a very small distance element $dx$ that is at distance $x$ from centre point $O$ of the earth then the work done to move an object from point $B$ to $A$

$dw=F.dx$

Now substitute the value of $F$ from equation $(1)$ in above equation

$dw=G \frac{M_{e} \: m}{x^{2}}.dx$

Therefore, the work done to bring the object from infinity to point $P$ that is at distance $r$ from centre point $O$ of the earth then the work done to move an object from infinity ($\infty$) to point $P$

$\int_{0}^{W} dw = \int_{\infty}^{r} G \frac{M_{e} \: m}{x^{2}}.dx $

$ [w]_{0}^{W} = G\: M_{e}\: m \int_{\infty}^{r} \frac{1}{x^{2}}.dx $

$ [W-0] = G\: M_{e}\: m [-\frac{1}{x} ]_{\infty}^{r} $

$ W = G\: M_{e}\: m [\left(-\frac{1}{r}\right) - \left(-\frac{1}{\infty}\right)]$

$ W = -\frac{G\: M_{e}\: m }{r} \qquad \left( \because \frac{1}{\infty} =0 \right)$

$ W = -\frac{G\: M_{e}\: m }{r} $

$\frac{W}{m} = -\frac{G\: M_{e} }{r} $

$ V = -\frac{G\: M_{e}}{r} \qquad \left( \because V=\frac{W}{m} \right)$

$ V = -\frac{G\: M_{e}}{r}$

Where $r=R_{e}+h$, then above equation can be written as

$V=-\frac{G \: M_{e}}{R_{e}+h} \qquad(2)$

This is the equation of gravitational potential at point $P$. The other form of the above equation i.e

$V=-\frac{g R_{e}^{2}}{R_{e}+h} \qquad \left( \because GM_{e}= g R_{e}^{2} \right)$

If the object is placed on the surface of the earth then $h=0$. So gravitational potential on the surface of the earth

$V=-\frac{G \: M_{e}}{R_{e}}$

This is the equation of gravitational potential on the surface of the earth. The other form of the above equation is

$V=-\frac{g R_{e}^{2}}{R_{e}} \qquad \left( \because GM_{e}= g R_{e}^{2} \right)$

$V=-g R_{e}$