Definition and Practical Applications of Centripetal Force

Definition of Centripetal Force:

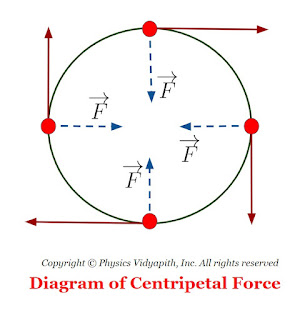
When a particle moves in a circular path then a force act, toward the centre of the circle, on a particle. This type of force is called the centripetal force.

This force is also known as a radial force because the direction of force is toward the centre of the circle.

Let us consider, A particle of mass $m$ moving around a circular path of radius $r$ with linear velocity $v$. So the force on a particle:

$F=ma$

Where $a$ is centripetal acceleration i.e. $a=\frac{v^{2}}{r}$. So the force on a particle can be written as

$F=m\frac{v^{2}}{r}$

$F=\frac{mv^{2}}{r}$

$F=m\frac{(r \omega)^{2}}{r} \qquad \left( \because v=r \omega \right)$

$F=mr\omega^{2}$

$F=mr\left(\frac{2\pi}{T}\right)^{2} \qquad \left( \because \omega=\frac{2\pi}{T} \right)$

$F=mr\frac{4\pi^{2}}{T^{2}}$

$F= \frac{4 \pi^{2} m r}{T^{2}} $

$F=4 \pi^{2} m r n^{2} \qquad \left( \because n=\frac{1}{T} \right)$

Practical Applications of Centripetal Force:

1. The planets revolve around the sun and the necessary centripetal force is achieved by gravitational force between the sun and the planet. as shown in the figure below

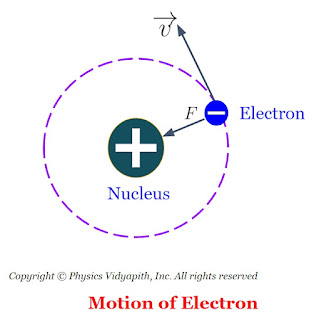
2. The electrons revolve around the nucleus of the atom and the necessary centripetal force is achieved by the force of attraction on the revolving electrons and protons in the nucleus. as shown in the figure below

3. In this case, When a piece of stone is tied to the end of a string whose other end is held in the hand then the required centripetal force, to revolve the stone in a circular path, is achieved by the tension of the string.

4. When a motor car is moving in a curved path then the necessary centripetal force is achieved by friction between the wheels and the road.

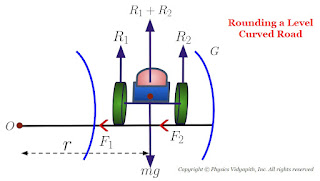
5. Rounding a Level Curve Road: When a vehicle goes in round a curved road, it requires some centripetal force. While rounding the curve, the wheels of the vehicle have a tendency to leave the curved path and regain the straight line path. The force of friction between the wheels and the road opposes this tendency of the wheels. This frictional force acts towards the centre of the circular track and provides the required centripetal force.

The forces acting on moving vehicles on the curved road are

Weight $mg$ of the vehicle act vertically downward

Normal reaction $R_{1}$ and $R_{2}$ from both sides of the wheels act vertically upward from the horizontal surface

The frictional force $F_{1}$ and $F_{2}$ from both sides of wheels of the vehicle

Hence, the centripetal force is provided by friction force only, therefore

$\frac{mv^{2}}{r} \leq F_{1}+F_{2}$

Where $F_{1}=\mu R_{1}$ and $F_{2}=\mu R_{2}$, then

$\frac{mv^{2}}{r} \leq \mu R_{1}+\mu R_{2}$

$\frac{mv^{2}}{r} \leq \mu \left( R_{1}+ R_{2} \right)$

$\frac{mv^{2}}{r} \leq \mu R \qquad \left( R= R_{1}+ R_{2} \right)$

$\frac{mv^{2}}{r} \leq \mu mg \qquad \left(R=mg \right)$

$\frac{v^{2}}{r} \leq \mu g $

$v^{2} \leq \mu r g$

$v \leq \sqrt{\mu r g}$

Here, $\mu$ is the frictional coefficient.

This is the maximum velocity of the vehicle for rounding on a level curved road without sliding. But the value is very low as it depends on the friction coefficient.

6. Banking of roads: The phenomenon of raising the outer age of the curved road above the inner age is called the banking of roads. When a vehicle moves on the curved path at a reasonable speed without slipping then sufficient centripetal force is required to move in a curved path which is achieved by banking of the road.

In the case of the Banking of roads, The centripetal force is not provided by friction between the wheels and the road.

Calculation of the angle of banking:

Let us consider, A vehicle of mass $m$ moving around the curved path of radius $r$ with velocity $v$ on a road banking through an angle $\theta$. The forces applying to the vehicle are:

A.) The weight $mg$ act vertically downward.

B.) Normal reaction $R$ of the ground acts perpendicular to the banked road and can be resolved into two components. i.e. Verticle component $R sin\theta$ and Horizontal component $R cos\theta$.

C.) The horizontal component $R cos\theta$ is balanced by the weight $mg$ of the vehicle. i.e.

$R cos\theta= mg \qquad (1)$.

D.) The vertical component of normal reaction $R sin\theta$ acts along the horizontal towards the centre of the circular path and it provides the centripetal force. i.e.

$R sin\theta=\frac{mv^{2}}{r} \qquad (2)$

Now divide equation $(2)$ and equation $(1)$

$\frac{R sin\theta}{R cos\theta}= \frac{\frac{mv^{2}}{r}}{mg}$

$\frac{ sin\theta}{ cos\theta}=\frac{v^{2}}{rg} $

$ tan\theta=\frac{v^{2}}{rg} \qquad(3)$

$ \theta=tan ^{-1} \left( \frac{v^{2}}{gr} \right)$

If $h$ is the height $AB$ of the outer edge of the road above the inner edge and $b$ is the breadth $OB$ of the road then from the figure:

$OA=\sqrt {(OB)^{2}-(AB)^{2}}$

$OA=\sqrt {b^{2}-h^{2}}$

$tan\theta=\frac{AB}{OA}$

$tan\theta=\frac{h}{\sqrt {b^{2}-h^{2}}} \qquad (4)$

From equation $(3)$ and equation $(4)$

$tan\theta=\frac{v^{2}}{rg}=\frac{h}{\sqrt {b^{2}-h^{2}}}$

From the above equation, we can calculate $h$. Usually $h << b$. Therefore, $h^{2}$ is negligibly small compared to $b^{2}$. The above equation can be written as:

$tan\theta=\frac{v^{2}}{rg}=\frac{h}{b}$

Roads are usually banked for the average speed of the vehicle passing over them. However, If the speed of a vehicle is somewhat less or more than this, the self-adjusting static friction will operate between the tyres and the road, and the Vehicle will not skid.

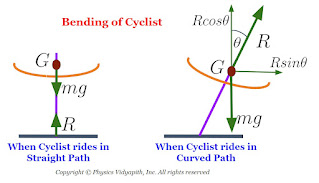
7. Bending of Cyclist: When a cyclist takes a turn on the curved path then the cyclist has to bend the cycle a little inwards from his vertical position to achieve the necessary centripetal force.

Calculation of the angle of bending:

Let us consider, The cyclist of mass $m$ moving around the curved path of radius $r$ with velocity $V$. The force acting on the cyclist:

A.) The weight $mg$ act vertically downward.

B.) Normal reaction $R$ of the ground act on the cyclist at an angle $\theta$ with the verticle and can be resolved into two components. i.e. Verticle component $R sin\theta$ and Horizontal component $R cos\theta$.

C.) The horizontal component $R cos\theta$ is balanced by the weight $mg$ of the cyclist. i.e.

$R cos\theta= mg \qquad (1)$.

D.) The vertical component $R sin\theta$ acts along the horizontal towards the centre of the circular path and it provides the centripetal force. i.e.

$R sin\theta=\frac{mv^{2}}{r} \qquad (2)$

Now divide equation $(2)$ and equation $(1)$

$\frac{R sin\theta}{R cos\theta}= \frac{\frac{mv^{2}}{r}}{mg}$

$\frac{ sin\theta}{ cos\theta}=\frac{v^{2}}{gr}$

$ tan\theta=\frac{v^{2}}{gr}$

$ \theta=tan ^{-1} \left( \frac{v^{2}}{gr} \right)$