Capacitance of a Parallel Plate Capacitor Partly Filled with Dielectric Slab between Plates
Derivation→
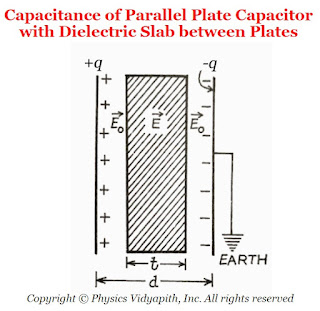
Let us consider,
The charge on a parallel-plate capacitor = $q$
The area of parallel-plate = $A$
The distance between the parallel-plate = $d$
The dielectric constant of the slab of a material =$K$
The thickness of the material =$t$
The vacuum (or air) between the plates =$(d-t)$ The surface charge density on the plates= $\sigma$
The electric field in the air between the plates is
$E_{\circ}=\frac{\sigma}{\epsilon_{\circ}}$
$E_{\circ}=\frac{q}{\epsilon_{\circ}\: A} \qquad(1)$
The electric field in the dielectric material
$E=\frac{q}{\epsilon_{\circ}\: K\: A} \qquad(2)$
The potential difference between the plates
$V=E_{\circ}\left( d-t \right)+E\:t \qquad(3)$
Now substitute the value of $E_{\circ}$ and $E$ in the equation $(3)$, Then we get
$V=\frac{q}{\epsilon_{\circ}\: A} \left( d-t \right)+ \frac{q}{\epsilon_{\circ}\: K\: A} \:t $
$V=\frac{q}{\epsilon_{\circ}\: A} \left[ \left( d-t \right)+ \frac{t}{K} \right] \qquad$
The capacitance of the capacitor is
$C=\frac{q}{V}$
Now substitute the value of electric potential $V$ in the above equation then
$C= \frac{q}{\frac{q}{\epsilon_{\circ}\: A} \left[ \left( d-t \right)+ \frac{t}{K} \right]}$
$C= \frac{\epsilon_{\circ}\: A}{\left[ \left( d-t \right)+ \frac{t}{K} \right]} $
Since $K>1$, the 'effective' distance between the plates becomes less than $d$ and so the capacitance increases.
Special Cases→
The area of parallel-plate = $A$
The distance between the parallel-plate = $d$
The dielectric constant of the slab of a material =$K$
The thickness of the material =$t$
The vacuum (or air) between the plates =$(d-t)$ The surface charge density on the plates= $\sigma$
 |
| The capacitance of Parallel Plate Capacitor with Dielectric Slab between Plates |
- When the dielectric slab is completely filled between the parallel plates i.e. $t=d$ then the capacitance between the parallel plates
$C= \frac{K \: \epsilon_{\circ}\: A}{d}$
- When there is a vacuum (or air) between the parallel plates i.e. $t=0$, then the capacitance between the parallel plates
$C_{\circ}= \frac{ \epsilon_{\circ}\: A}{d}$
- When there is a slab of metal whose dielectric constant is infinity (K=∞). If the thickness of the slab is t between the parallel plates, then the capacitance between the parallel plates
$C= \frac{ \: \epsilon_{\circ}\: A}{d-t}$
- When the slabs of dielectric constants $K_{1},K_{2},K_{3},K_{4}...........$ and respective thickness $t_{1},t_{2},t_{3},t_{4},........$ is placed in the entire space between the parallel-plates, then the capacitance between the plates
$C=\frac{\epsilon_{\circ} A}{d- \left( t_{1}+t_{2}+t_{3}+.... \right)+ \left( \frac{t_{1}}{K_{1}}+\frac{t_{2}}{K_{2}}+\frac{t_{3}}{K_{3}}+....... \right)}$But $d=t_{1}+t_{2}+t_{3}+....$$C=\frac{\epsilon_{\circ} A}{ \left( \frac{t_{1}}{K_{1}}+\frac{t_{2}}{K_{2}}+\frac{t_{3}}{K_{3}}+..... \right)}$

